Definition
The original variation between the actual value and the calculated value extracted in the form of percentage is termed to be the percentage error. This tool is used to measure whether the data collection is progressing in the right direction and is mostly used by corporate companies and statistic experts. If taken the context of academic career, the percentage error formula is very crucial for the students pursuing their degrees in the discipline of economics and science. While conducting a study or calculations from the figures provided in a database, various errors could occur.

Majorly the percentage error formula is used to determine how accurate the calculated value is by keeping in mind the actual value. I mentioned in a very rough language, it is the variation of the gap between the calculated value and the genuine value expressed in the form of a percentage. Commonly, the extent of the percentage error is expressed in the positive denominations, although having a negative percent error is not a wrong approach. Relying on the sign of the percentage error measure, it could be analyzed whether the calculated value is being paced below or higher to the actual value. The tool of percentage error formula is one of the most reliable and accurate tools in calculating the relative error by keeping the original measure as the basis.

While calculating the database of a certain type, normally three types of errors occur while dealing with it. They are: –

• Human errors.
• Random errors.
• Systematic errors.

Human errors
It is the mistake that happens because of the poor management and calculation from behalf of the human resources. In layman’s language, this sort of error could also be termed as blunders. Let us take the instance that an official has entered the figures in a wrong manner by taking in account wrong units or conducting some performance type of issues, etc.

Random errors
This sort of mistake comes under the category of Random errors. These errors come under the section, which occurs because of the external disturbances like high noise, performance issues, lack of rest, inconvenient working environment, etc.

Systematic errors
It is the mishandling of the measuring parameters which leads the way to this sort of errors. If the instruments are not properly synchronized, or the employees are not trained to handle that tool then it is quite evident that the systematic errors would occur in that instance. The errors would result in the recording of wrong information.

The disparity in the observations
It is quite often observed that when the employees make use of two different tools for the same purpose, or if the same process is being done multiple times, the observations display a considerable limit of variations. This phenomenon is often termed as the disparity in observations or variation in the measurement. This substantial disparity is often termed as the error in the measurement. Although for the technical or the statistical purpose, this context is denoted by the term, uncertainty in the measurement.

If the instance of disparity in the measurement occurs, it generally signifies that the genuine and actual measurement differs from the calculated measures. From this context, it could be deducted that instead of conducting the process of calculation very meticulously and carefully, the errors may occur because of slight mishandling of the data. It is almost impossible to conduct an extensive impeccable calculation by avoiding all the mistakes. Hence it is evident that most probably errors would happen in the calculation. The percentage error formula would turn out to be a very significant tool in deciding the gap between the calculated measures and actual measures.

By using the below expression, you could measure the gap or the variation in the measurement. Discrepancy in measures = Actual Value – Calculated Value.

The precision of the Tools or the Instruments
It is because of the above-mentioned errors or lack of accuracy in the used tools or instruments these sorts of discrepancies happen in between the actual and calculated observations. By the term, precision, the efficiency of the tool to measure the smallest possible unit is signified.

Accuracy of the tools
By the term, the accuracy of the tools, the ability of the concerned tool to display the observation which is quite close to the original measurement.

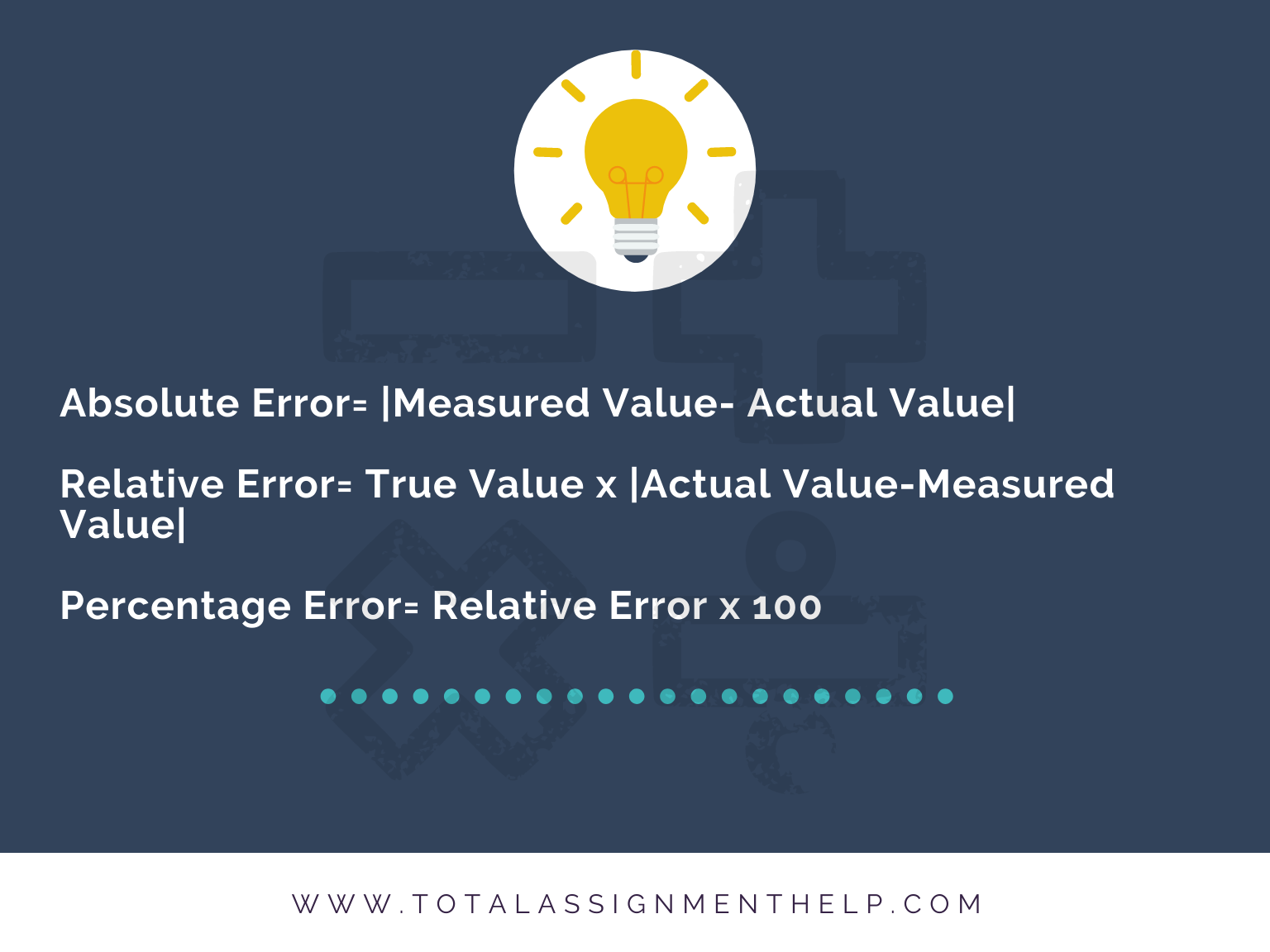
Absolute Error Formula
By using this formula, you could ascertain the level to which the physical error has happened in the calculation. By using a simple arithmetic formula, the physical error could be calculated. Below is provided the absolute error formula:

The absolute could be calculated by taking the backward approach using the values of relative error and actual value.

Relative Error By keeping the actual value as the basis, the absolute error is being calculated in the concept of relative error. The equation to find the relative error is provided below: –

The use of relative error is done to analyze the extent of accuracy in the calculation.

Percent Error Formula This formula also comes under the division of comparison formula. The measured observation is being compared with the actual value of the observation and the whole gap is represented in the form percentage difference. The percentage gap is represented by keeping the true value as the basis.

The tool of the percent error formula comes under the classification of the approximation error. The tools which come under the category of approximation generally would showcase the gap existing in between the calculated value and the actual value. The errors in the classification generally occur because of factors like deficiency of precision and accuracy. For instance, instead of using the accurate value of π, implying just 3.14 as the value would bring potential and calculative errors in the observation.

The product of the relative error with the integer 100 would provide the percentage error and is the basic methodology in the percentage error formula. The mathematical expression for the percentage error is provided below: –

The way to imply Percentage error formula
To correctly imply the percentage error formula, follow the below-given suggestions.

Find out the difference in between the values of the actual and the calculated measures. You don’t have to bother about the sign of the obtained measure. The actual goal is to find the percent error by using the percentage error formula and hence the negative sign does not make much impact in the calculation. The resulting figures should be divided by the actual observation and the resulting figure most probably would be a decimal number. The resulting number should be then multiplied with 100 to obtain the original value of percent error. The error could be calculated very easily by using the tool of percentage error formula.

Let’s take an example of a cylindrical prism. To measure various dimensions of the cylinder, it is most possible that you would use the Vernier calipers. Assume that the measured length of the cylinder is 2.68 mm. Though the actual length of the cylinder is 2.70 mm. The following are the steps to be followed in calculating the concerned error by using the percent error formula.
Percent error = (2.70 – 2.68270) x 100 %
= 0.02270 x 100 % = 0.74 %

Thus, the Vernier calipers displays the measurement with the variation of 0.74% from the actual measurement.

You could also refer to some of the other formulae to find out the measure of percentage error in the existing calculation. Which are listed below?
Real measurement = Measured one + percent error x 100 %
Real measurement = Measured one – percent error x 100 %

Note: Since the determinant or the absolute value is being considered in this approach, it is quite probable that the actual measurements may possess two different values. The calculated values could be obtained by following the below-listed formulae.

Calculated Value = (Real measure + percent error) x 100 %
Calculated Value = (Real measure – percent error) x 100 %

You could also use a different percentage error formula in calculating the error. It could be represented as the below-given equation:

Percentage error = (measure 1 + measure 2) (measure 1 – measure 2) x 100 %

Problem 1
It was being observed while experimenting with a person that the boiling point of a substance is 54.9 degrees Celsius. Although it is a commonly known fact that the actual boiling point of the substance is 54.0 degree Celsius. From the given data, calculate the measure or the extent of absolute error, percentage error, and the relative error by implying the percentage error formula.

Answer
Percentage error = (54.9 – 54.054) x 100 = 2 %
Absolute error = 54.9 – 54.0 = 0.9
Relative error = 54.9 – 54.054 = 0.02

Problem 2
While experimenting, a student has observed the molecular mass of the bromine to be 36.3. Although the real mass of the bromine is recorded to be 36.2. By using the aforementioned data, calculate the relative error, percentage error, and the absolute error by implying the percentage error formula.

Answer
Percent error = 0.0276 x 100 % = 2.76%
Relative error = 0.136 x 2 = 0.0276
Absolute error = 36.3 – 36.2 = 0.1

Problem 3
The real provided length of the wire is proved to be 3.53 m along with specifying that the gap of percentage error would be 5%. By using the provided details, calculate the length of the wire the person could have calculated.

Answer
Calculated value = (Real value + percent error) x 100 %
Calculated value = (3.53 + 5 %) x (3.53100 %) = 3.71 m
Otherwise
Calculated Value = (Real value – PERCENT ERROR) x 100
Calculated Value = (3.53 – 5 %) x (3.53100 %) = 3.35 m.

Problem 4
While experimenting, it was found that the inner diameter of a hollow cylinder turns out to be 8.03 mm. Since it is provided that the percent error is 4% systematically calculate the real value by using the percentage error formula.

Solution
Real measure = PERCENTAGE ERROR + MEASURED 1
Real Value = 8.031 + 40% = 7.72

OR
Actual Value = MEASURED 1 + PERCENTAGE ERROR
Actual Value = 8.031 – 40 % = 7.71

Problem 5
In a concert conducted, it was observed that around the total sum of 70 people has attended it. Although it was a fact that 80 people had booked their seats and have attended the concert. By using the provided value, calculate the percent error that happened in the appropriation of the audience by utilizing the percentage error formula.

Solution:
Relative error = 10 / 80 = 0.125
Percent error = 0.125 x 100 = 12.5 %
Absolute error = 80 – 70 = 10.

If the negative sign is considered in mind the subtraction, the level of the error could be appropriated and could be situated above or below the real value.

Problem 6
A ball takes around the distance of 2 m to come down to the ground, after been thrown by the student. The time recorded by the ball to land was around 0.62 s. Although it was observed that the actual time taken by the ball to land by considering the equations of motion is 0.64 seconds. By implementing the percentage error formula calculate the percent error.

Answer
Percent error = 100 x (Calculated Value – Real value)
= 0.62 – (0.64 x 0.64) x 100 = -3.12%.

Hence, the calculated reading was observed to be below by the range of 3.12% as compared to the real value.

Problem 7
It was being predicted by the forecast and weather department that a particular section of the area would get the rainfall of around 20 mm. Although the actual rainfall had reached a measure of 25 mm. By using the percentage error formula, please calculate the percentage error in this context.

Answer
As the equation states,
Percent of error = Calculated figure – Real value True Value x 100 %
Percentage error = 25 – 2020 x 100 % = 2.5 %
Hence it could be concluded that the gap between the original rainfall and the calculated rainfall sums up to 2.5 %.